Friday, September 09, 2011

Shivaji Ganesan

Tuesday, November 23, 2010

clock puzzles

Some interesting maths about clock

When do the hands of the clock line up?

When do they form equal angles? When do they point in opposite directions?

The hour & minute hands align perfectly every 43200/11 seconds
(1 hour, 5 minutes, 27.273 seconds).
This happens 11 times every 12 hours.
Exactly in between the times they align perfectly, they are at
exact opposition (11 times every 12 hours).
The hour & second hands align perfectly every 43200/719 seconds
(60.083 seconds).
This happens 719 times every 12 hours.
Exactly in between the times they align perfectly, they are at
exact opposition (719 times every 12 hours).
The minute & second hands align perfectly every 3600/59 seconds
(61.017 seconds).
This happens 708 times every 12 hours.
Exactly in between the times they align perfectly, they are at
exact opposition (708 times every 12 hours).
The hour hand bisects the angle between the minute & second hands
every 4320/73 seconds (59.178 seconds).
This happens 730 times every 12 hours.
The minute hand bisects the angle between the hour & second hands
every 43200/697 seconds (61.980 seconds).
This happens 697 times every 12 hours.
The second hand bisects the angle between the hour & minute hands
every 43200/1427 seconds (30.273 seconds).
This happens 1427 times every 12 hours.

The last three data files also include the angle that is made by the
bisecting hand. We also consider it a bisection when the bisector splits
the "back" of the angle (i.e, splits the > 180 degree angle side).

Twelve o'clock is included in the counts of 1, 3, 5, 7, 8, 9.
Six o'clock is included in the counts of 2, 4, 5, 7.
The rest of the times are all unique, giving 5723 total "interesting" times
on the clock.

You may also consider "interesting" the times when two clock hands form
a 90-degree angle. This happens exactly in between when a pair of hands
align and when they oppose, so it happens 22 times for H&M, 1438 times
for H&S, and 1416 times for M&S. Note that three o'clock and nine o'clock
are counted multiple times, so this brings the total interesting times
to 8595.

How did you calculate these values?

The math for this is fairly elementary. First of all, let's make the
following observations:

The second hand moves 6 degrees every second.
The minute hand moves 1/10 degree every second.
The hour hand moves 1/120 degree every second.

So if t seconds have elapsed since 12 o'clock, the hands have moved
6t, t/10, and t/120 degrees, respectively. We will need to interpret these
degrees mod 360.

Now, let's say we want to find the times where the hour hand (H) is exactly between
the minute (M) and second (S) hands. This means that the angular distance from M to H is the same as the distance from H to S. In other words:

M - H = H - S (mod 360)

Simplifying this, we get that M-2H+S must be a multiple of 360. If we
substitute in S=6t, M=t/10, and H=t/120, we get that:

t/10 - t/60 + 6t = (73/12)t is a multiple of 360

In other words, t must be a multiple of 360*(12/73) = 4320/73. So the
hour hand bisects the other two every 4320/73 = 59.178 seconds, which is
exactly what happens.

The other calculations for when one hand is exactly between two other hands
are very similar and follow the same structure.

If we want to find out when two hands line up (say, the hour and second hands),
this is even easier. The two hands aligning simply means that

H = S (mod 360)

Or in other words, S-H is a multiple of 360. Substituting in, this means that

6t - t/120 = (719/120)t is a multiple of 360

So the alignment happens every 360*(120/719) = 43200/719 = 60.083 seconds,
which matches what
we calculated.

The minute hand and the hour hand exactly coincides at the following time in hour minute and seconds:

12:00:00.000
01:05:27.273
02:10:54.545
03:16:21.818
04:21:49.091
05:27:16.364
06:32:43.636
07:38:10.909
08:43:38.182
09:49:05.455
10:54:32.727

Sunday, January 31, 2010

IBM Grand Slam Widget

Sunday, December 09, 2007

magmypic

Create Fake Magazine Covers with your own picture at MagMyPic.com

Subscribe to National Geographic Magazine at a 30% discount!

The following animation shows the most famous of Lloyd picture puzzle where out of 13 chinese men perched on the top of the globe, one suddenly goes missing when the globe is slightly tilted. Where did he go?

Create Fake Magazine Covers with your own picture at MagMyPic.com

Monday, April 30, 2007

Hats Blown Off

Ten people, each wearing his own hat, are crossing a desert when suddenly a gust of wind blows and all the hats were blown off. That time a boy happens to cross nearby and he takes out all the hats and return to them. Now the question is what is the probability of exactly nine hats being returned to the right persons? (i.e) returning the hats to its original owners?

Answer:

The probability is Zero. If exactly 9 hats are returned to them correctly, so must be the 10th hat to the 10th person. It is not a question of probability; or rather, a certainty. There is no mathematics involved here; just a mere commonsense; that's all.

Volume of a Hole

If the earth is digged into a hole of 2 feet length, 2 feet breadth, and 2 feet depth, how much cubic feet of dirt is in the hole?

Answer:

If any one hastens to say it is 8 cubic feet, woebegone for intellectual vacuum! There is no dirt in the hole, as otherwise how can it be called a hole at all?

Sum of First 100 Natural Numbers

If the qustion of sum of first 100 natural numbers is asked most people will readily answer as 5050 using n(n+1)/2 formula. When the same question was asked to Carl Frederick Gauss, the greatest mathematician ever lived, when he was barely 5 years old, he solved it in a most natural and ingenious way for a 5 year old. This method is a basic idea for deriving n(n+1)/2. Can you just imagine how he did it?

Answer:

First he (Gauss) imagined that he had written all the first 100 Natural numbers and started adding in the following way:

1+2+3+4+5+................................97+98+99+100

= (1+100)+(2+99)+(3+98)+(4+97)+..................(50+51)

=101 + 101 + 101 + 101 +.................. 101

= 101 summed 50 times, (because 100 numbers are paired into 50)

= 101 x 50 = 5050.

Truly remarkable for a tender 5 year old to conceive and devise such a marvellous and wonderful method.

100 Vegetables for 100 Dollars

20 Brinjals cost $1; 1 cucumber costs $5; 1 coconut costs $1. You must purchase a combination of all these vegetables, the total number of vegetables should be 100 and also its cost should be $100. (i.e.) make a purchase of 100 vegetables whose cost is also $100.

Answer:

Most people would certainly lament over the fact that 3 unknowns are there but however, only 2 equations could be set-up and hence it cannot be solved. It is better to make an attempt before making any such decision. If we assign the required brinjals, cucumbers and coconuts as x, y, and z then we have

x/20 + 5y + z = 100 ...............(1)

x + y + z = 100 ...............(2)

(2)-(1) gives 19x/20 - 4y = 0.

19x/20 = 4y.

19x = 80y.

x/y = 80/19.

Since 80 and 19 are relatively prime to each other and also x+y+z=100 the least values of x and y should be 80 and 19 and therefore z=1. Hence the soultions are 80 brinjals,19 cucumbers and 1 coconut. Q.E.D.

Mysterious Suicide

An inspector breaks open a room, bolted from inside, finds a body of a man hanging from the ceiling. The dead man is too short a person to do the hanging from the ceiling and there is absolutely no other thing available except some sheet of water on the floor. How could he have committed suicide?

Answer:

'Well', another good puzzle. Have you ever wondered how come the presence of water inside with no containers? If your thinking mill grinds on this score, you could possibly find it out! To cut the long story short, the man committed suicide by climbing over a largepiece of 'ice' and reached the ceiling. After that the ice melted into sheet of water. Got it!!

Convey Message

There are 3 persons, a blind, a deaf, and a dumb respectively. On a certain ill-fated day, the deaf, outrages the modesty of the blindperson's wife and the whole episode was watched by the dumb. He however conveyed the message to the blind person. How could he have done it? The dumb cannot speak or if he manages to pass on the information through sign language, unfortunately, the blind cannot see it. However he succeeded in conveying the message to the blind and the question is how did he accomplish the task?

Answer:

It is a beautiful puzzle because the dumb cannot convey the message orally. If he tried the 'sign language' and did some gestures, the blind cannot 'see' it. What an unfortunate situation!! Yet, the dumb conveyed the news successfully to the blind person. 'Astonishing', well, he conveyed the message to the blind through 'BRAILLE' system.This is the only possible way to have been communicated.

Planting of Roses

If you plant 4 rose saplings in a row and likewise do the same in 5 such rows, how many saplings in total would you have planted in all? Please do not say 20 as answer as it is very trivial one. Is there any other possibility meeting all the criterions?

Answer:

It could also be 10 if the roses are planted in the shape of a star!

The Missing Dollar

Three friends, sharing a single room in a remote sea-side resort, make an advance payment of $10 each. While they are checking out, the owner of the lodging house charges $25 and ask the room-attendant to give back the balance $5 to them. But the dishonest person, pockets $2 himself, and gives back $1 to each one of them. Now every one of the three friends had given $10 originally and gets back $1, thereby incurring an expense of $27. Adding this to $2, pocketed by room-attendant, does not tally with $30. Why? Where does the dollar disappear?

Answer:

Actually the dollar is not missing or lost. Only our thinking mind is 'lost'. Since the $27 contains $25 as rent and $2 as pocketed by room-attendant, where does the question of adding again $2 with $27 and expect it to become $30? Now the following equations will make you more clear: $25(rent) + $2(pocketed amount) + $3 (amount got back) = $ 30 $27(rent+ pocketed amount) + $ 2(pocketed amount) SHOULD NOT = to $30 as we are adding the pocketed amount 2 times. Hence the confusion. Got it?

A mammoth product

What is the value of the product of 26 algebraical expressions (x-a)(x-b)(x-c)...........upto (x-z)where a, b, c, ............ z represent the english alphabets?

Answer:

The answer is more trivial than you think, but more harder than you imagine! Solution: The answer is, just a moment, hold your breath, 0. Youwonder how? Because out of 26 algebraical expressions, (x-x) is also one among them whose vaule is 0. Hence the product is 0.
===================================================================================
Inflation Index(India)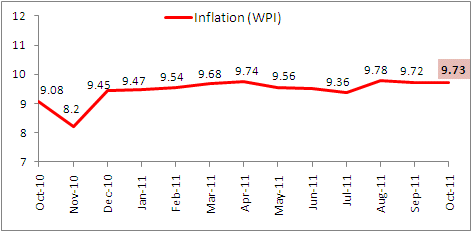

==================================================================================

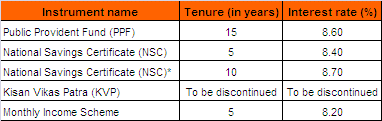
In this section we want to look at the graph of a quadratic function. The most general form of a quadratic function is,

The graphs of quadratic functions are called parabolas. Here are some examples of parabolas.

Parabolas_Vertex

Tuesday, October 05, 2004

Can you explain?

Two persons are travelling in a car at the rate of 45 kmph and suddenly the person driving the car stops in front of a shop to buy some items and after purchasing the same, reaches the car when, to his surprise and dismay find the other person dead and moreover a stranger is inside the car. The stranger had not entered the car before they started nor the second person who was dead let him in while the first one went to the shop. What is the possible and logically correct explanation you can give?

Answer:

The two person were a man and his wife, and the lady was on labour and so they were rushing to a hospital enroute a shop to purchase some pills and the woman delivered a child and herself passed away in the post-delivery complications.
=================================================================================
Walk and ride: 2 pedestrians have one bike. They travel as follows: the first one rides the bike for some time, then drops it and continues walking in the same direction, while the second follows him by foot. Eventually, the second one finds the bike and rides it until he overtakes the first one. Then he gives the bike to the first one etc. Assuming that they walk with the same speed and bike faster than they walk, what is the speed of their travel?

Ans: The answer is: the harmonic average (the reciprocal of the arithmetic average of the reciprocals) of the walk and bike speeds. This is because both walk and bike equal distances.
===================================================================================

A few photos of Bobby Fischer

a pictorial form of "Sieve of Erotosthenes"

The Euler product formula for the Riemann zeta function reads

$\sum_{n=1}^\infty\frac{1}{n^s} = \prod_{p \text{ prime}} \frac{1}{1-p^{-s}}$

where the left hand side equals the Riemann zeta function:

$\zeta(s) = \sum_{n=1}^\infty\frac{1}{n^s} = 1+\frac{1}{2^s}+\frac{1}{3^s}+\frac{1}{4^s}+\frac{1}{5^s}+ \ldots$

and the product on the right hand side extends over all prime numbers p:

$\prod_{p \text{ prime}} \frac{1}{1-p^{-s}} = \frac{1}{1-2^{-s}}\cdot\frac{1}{1-3^{-s}}\cdot\frac{1}{1-5^{-s}}\cdot\frac{1}{1-7^{-s}} \cdots \frac{1}{1-p^{-s}} \cdots$

[edit] Proof of the Euler product formula

The method of Eratosthenes used to sieve out prime numbers is employed in this proof.

This sketch of a proof only makes use of simple algebra that most high school students can understand. This was originally the method by which Euler discovered the formula. There is a certain sieving property that we can use to our advantage:

$\zeta(s) = 1+\frac{1}{2^s}+\frac{1}{3^s}+\frac{1}{4^s}+\frac{1}{5^s}+ \ldots$

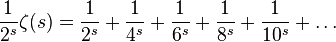
$\frac{1}{2^s}\zeta(s) = \frac{1}{2^s}+\frac{1}{4^s}+\frac{1}{6^s}+\frac{1}{8^s}+\frac{1}{10^s}+ \ldots$

Subtracting the second from the first we remove all elements that have a factor of 2:

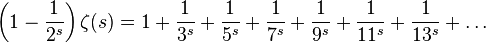
$\left(1-\frac{1}{2^s}\right)\zeta(s) = 1+\frac{1}{3^s}+\frac{1}{5^s}+\frac{1}{7^s}+\frac{1}{9^s}+\frac{1}{11^s}+\frac{1}{13^s}+ \ldots$

Repeating for the next term:

$\frac{1}{3^s}\left(1-\frac{1}{2^s}\right)\zeta(s) = \frac{1}{3^s}+\frac{1}{9^s}+\frac{1}{15^s}+\frac{1}{21^s}+\frac{1}{27^s}+\frac{1}{33^s}+ \ldots$

Subtracting again we get:

$\left(1-\frac{1}{3^s}\right)\left(1-\frac{1}{2^s}\right)\zeta(s) = 1+\frac{1}{5^s}+\frac{1}{7^s}+\frac{1}{11^s}+\frac{1}{13^s}+\frac{1}{17^s}+ \ldots$

where all elements having a factor of 3 or 2 (or both) are removed.

It can be seen that the right side is being sieved. Repeating infinitely we get:

$\ldots \left(1-\frac{1}{11^s}\right)\left(1-\frac{1}{7^s}\right)\left(1-\frac{1}{5^s}\right)\left(1-\frac{1}{3^s}\right)\left(1-\frac{1}{2^s}\right)\zeta(s) = 1$

Dividing both sides by everything but the $ζ(s)$ we obtain:

$\zeta(s) = \frac{1}{\left(1-\frac{1}{2^s}\right)\left(1-\frac{1}{3^s}\right)\left(1-\frac{1}{5^s}\right)\left(1-\frac{1}{7^s}\right)\left(1-\frac{1}{11^s}\right) \ldots }$

This can be written more concisely as an infinite product over all primes p:

ζ(s) =	∏	(1 − p ^{− s}) ^{− 1}.
	p

To make this proof rigorous, we need only observe that when Re(s) > 1, the sieved right-hand side approaches 1, which follows immediately from the convergence of the Dirichlet series for $ζ(s)$ .

An interesting result can be found for $ζ(1)$

$\ldots \left(1-\frac{1}{11}\right)\left(1-\frac{1}{7}\right)\left(1-\frac{1}{5}\right)\left(1-\frac{1}{3}\right)\left(1-\frac{1}{2}\right)\zeta(1) = 1$

which can also be written as,

$\ldots \left(\frac{10}{11}\right)\left(\frac{6}{7}\right)\left(\frac{4}{5}\right)\left(\frac{2}{3}\right)\left(\frac{1}{2}\right)\zeta(1) = 1$

which is,

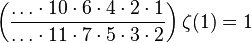
$\left(\frac{\ldots\cdot10\cdot6\cdot4\cdot2\cdot1}{\ldots\cdot11\cdot7\cdot5\cdot3\cdot2}\right)\zeta(1) = 1$

as, $\zeta(1) = 1+\frac{1}{2}+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+ \ldots$

thus,

$1+\frac{1}{2}+\frac{1}{3}+\frac{1}{4}+\frac{1}{5}+ \ldots = \left(\frac{\ldots\cdot11\cdot7\cdot5\cdot3\cdot2}{\ldots\cdot10\cdot6\cdot4\cdot2\cdot1}\right)$

An elegant way to prove Square root of 2 is irrational.

If √2 were rational, we could write it as a fraction a/b in lowest terms. Then
a2=2b2.(Please read a squared = 2 x b squared
Look at the last digit of a2. It has to be 0, 1, 4, 5, 6 or 9. Now look at the last digit of 2b2. It has to be 0, 2 or 8. As a2 and 2b2 are the same number, its last digit must be 0. But that's only possible if a ends in 0 and b ends in 0 or 5. Either way both a and b are multiples of 5 contradicting a/b being in lowest terms.
==================================================================================

======================================================================

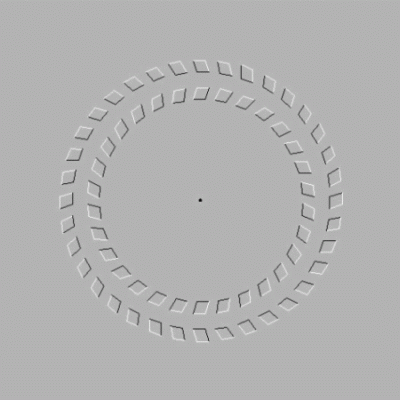Stare at the dot and slowly move your head towards the centre. What happens? Mystifying?
==================================================================================

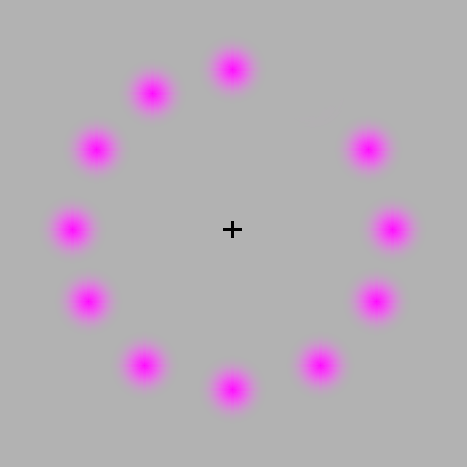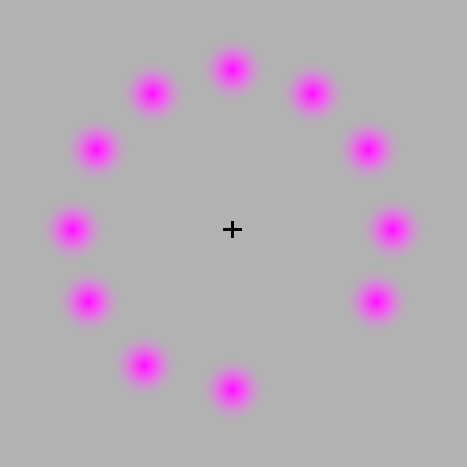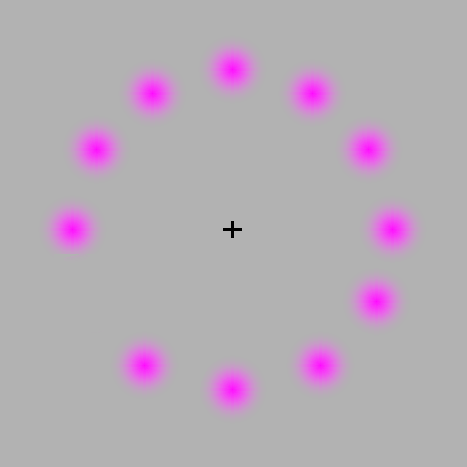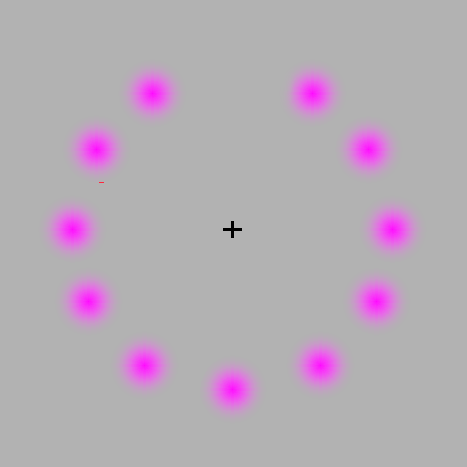Follow the movement of the rotating pink dot with your eyes and the dots will remain only one color, pink. But if you stare at the black + in the center, the moving dot will turn green.
================================================================================

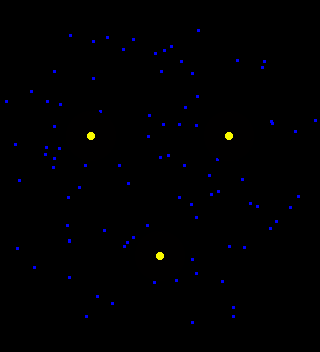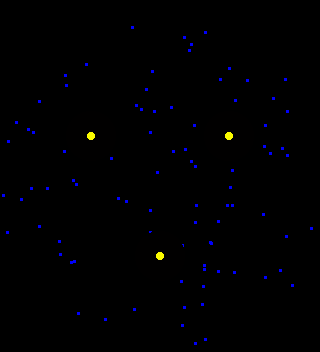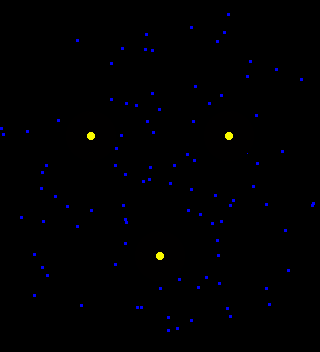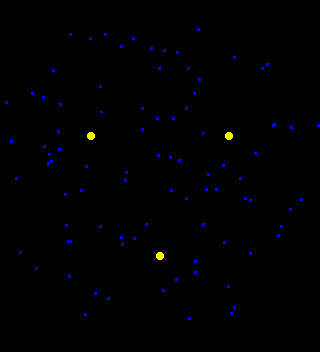If you stare at one yellow dot for a while, the other yellow dots disappear.
=============================================================================

From the picture cut out the six dismembered portions and try to set up to bring a complete normal horse.
The Answer

===================================================================================
Watch tcworks2's Free Webcam
Watch ainuralm's Free Webcam
Watch pangaea2's Free Webcam

For those who are interested in seeing Nava Brindavana photos:
click here

In the following diagram of matchsticks denoting 4 cubes, remove just one matchstick to make it 3.

Answer: remove the back of the middle cube in the second row
Solution

Answer

In the following diagram, how many times the area of the bigger square when compared to the smallest square?

Answer

How will you take the Tennis ball out of trench shown in the following diagram?

Answer

Picturial proof for area of the inside triangle

Age puzzle

Answer:They are of the same age Proof:

Age of mom and dad

This happened when the mother was 1 year old
now the mother's age is 43

free stock charts from in.advfn.com

==================================================================================
Watch hetij's Free Webcam
Watch eugeap's Free Webcam
=======================================================================================

Can you explain?

Friday, September 09, 2011

Shivaji Ganesan

Tuesday, November 23, 2010

clock puzzles

When do the hands of the clock line up?

How did you calculate these values?

Sunday, January 31, 2010

IBM Grand Slam Widget

Sunday, December 09, 2007

magmypic

Monday, April 30, 2007

Hats Blown Off

Volume of a Hole

Sum of First 100 Natural Numbers

100 Vegetables for 100 Dollars

Mysterious Suicide

Convey Message

Planting of Roses

The Missing Dollar

A mammoth product

Tuesday, October 05, 2004

Can you explain?

[edit] Proof of the Euler product formula

About Me

Previous Posts

Archives